-
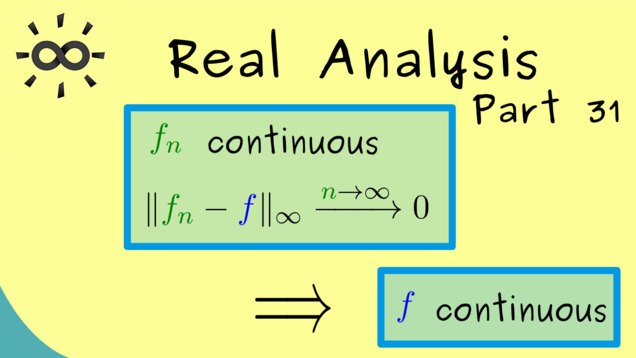
Title: Uniform Limits of Continuous Functions are Continuous
-
Series: Real Analysis
-
Chapter: Continuous Functions
-
YouTube-Title: Real Analysis 31 | Uniform Limits of Continuous Functions are Continuous
-
Bright video: https://youtu.be/llJruZnO-t4
-
Dark video: https://youtu.be/YUrHoTnMBhY
-
Ad-free video: Watch Vimeo video
-
Quiz: Test your knowledge
-
Dark-PDF: Download PDF version of the dark video
-
Print-PDF: Download printable PDF version
-
Exercise Download PDF sheets
-
Thumbnail (bright): Download PNG
-
Thumbnail (dark): Download PNG
-
Subtitle on GitHub: ra31_sub_eng.srt missing
-
Timestamps
00:00 Intro
00:14 Uniform convergence for sequence of functions
01:09 Theorem for uniform limit of continuous functions
02:18 Proof of the Theorem
07:41 Credits
-
Subtitle in English (n/a)
-
Quiz Content
Q1: Let $(f_1, f_2, f_3, \ldots)$ be a sequence of functions $f_n: I \rightarrow \mathbb{R}$. What is the correct definition for the uniform convergence to a function $f: I \rightarrow \mathbb{R}$?
A1: $ \displaystyle \forall x \in I ~~ \forall \varepsilon > 0 ~~ \exists N \in \mathbb{N} ~~ \forall n \leq N ~ : ~ |f_n(x)-f(x)|<\varepsilon $
A2: $ \displaystyle \forall \varepsilon > 0 ~~ \exists N \in \mathbb{N} ~~ \forall n \geq N ~~ \forall x \in I ~ : ~ |f_n(x)-f(x)|<\varepsilon $
A3: $ \displaystyle \forall x \in I ~~ \forall \varepsilon > 0 ~~ \exists N \in \mathbb{N} ~~ \forall n \geq N ~ : ~ |f_n(x)-f(x)|<\varepsilon $
A4: $ \displaystyle \forall \varepsilon > 0 ~~ \exists N \in \mathbb{N} ~~ \forall x \in I ~~ \forall n \leq N ~ : ~ |f_n(x)-f(x)|<\varepsilon $
Q2: Let $f_n: \mathbb{R} \rightarrow \mathbb{R}$ be continuous for all $n \in \mathbb{N}$. Which of the following cases can not occur?
A1: $(f_1, f_2, f_3, \ldots)$ is pointwisely convergent to $f$ and $f$ is not continuous.
A2: $(f_1, f_2, f_3, \ldots)$ is pointwisely convergent to $f$ and $f$ is continuous.
A3: $(f_1, f_2, f_3, \ldots)$ is uniformly convergent to $f$ and $f$ is continuous.
A4: $(f_1, f_2, f_3, \ldots)$ is uniformly convergent to $f$ and $f$ is not continuous.
Q3: Let $f_n: \mathbb{R} \rightarrow \mathbb{R}$ be continuous for all $n \in \mathbb{N}$ and $(f_n)_{n \in \mathbb{N} }$ be pointwisely convergent to $f$ given by: $$ \displaystyle f(x) = \begin{cases} 1 &, ~~ x = 0\ 0 &, ~~x \neq 0\end{cases} $$ Which statement is true?
A1: $| f - f_n |_\infty \xrightarrow{n \rightarrow \infty} 0 $
A2: The $(f_n)_{n \in \mathbb{N} }$ is not uniformly convergent to $f$.
A3: The $(f_n)_{n \in \mathbb{N} }$ is uniformly convergent to $f$.
-
Last update: 2025-01